Contents

Page updated: May 19, 2021
Author: Curtis Mobley
View PDF

The Luminance Transfer Equation

The Radiative Transfer Chapter derived the scalar radiative transfer equation (SRTE), Eq. (3) of the Scalar Radiative Transfer Equation page:

\begin{align} cos 𝜃 \frac{d L (z, 𝜃, ϕ, λ)}{d z} = & - c (z, λ) L (z, 𝜃, ϕ, λ) \\ + & \int_{0}^{2 π} \int_{0}^{π} β (z; 𝜃^{'}, ϕ^{'} \to 𝜃, ϕ; λ) L (z, 𝜃^{'}, ϕ^{'}, λ) sin 𝜃^{'} d 𝜃^{'} d ϕ^{'} \\ + & S (z, 𝜃, ϕ, λ) . & (1) \end{align}

This equation governs the propagation of unpolarized monochromatic radiance at a particular wavelength

λ

in a one-dimensional absorbing and scattering medium.

The question now arises: Is there a similar equation for the propagation of luminance? It is to be anticipated that a luminance transfer equation may be more complicated than the SRTE because it of necessity must involve all visible wavelengths and the response of the human eye.

The Luminance Transfer Equation

To develop a luminance transfer equation, multiply Eq. (1) by the photopic luminosity function $K_{m} \bar{y} (λ)$ and integrate over all visible wavelengths. Let $Λ$ denote the range of wavelengths for which $\bar{y} (λ) > 0$ . The term on the left hand side of the SRTE then becomes

K_{m} \int_{Λ} \{cos 𝜃 \frac{d L (z, 𝜃, ϕ, λ)}{d z}\} \bar{y} (λ) d λ = cos 𝜃 \frac{d L_{v} (z, 𝜃, ϕ)}{d z},

where the luminance $L_{v}$ is deﬁned by Eq. (1) of the Photopic Luminosity Function page:

L_{v} \equiv K_{m} \int_{Λ} L (λ) \bar{y} (λ) d λ .

The ﬁrst term on the right hand side of the SRTE becomes

K_{m} \int_{Λ} \{- c (z, λ) L (z, 𝜃, ϕ, λ)\} \bar{y} (λ) d λ .

This term does not give a product of an integral over wavelength of the beam attenuation coeﬃcient times the luminance. However, we can rewrite this term as

\{\frac{K_{m} \int_{Λ} - c (z, λ) L (z, 𝜃, ϕ, λ) \bar{y} (λ) d λ}{K_{m} \int_{Λ} L (λ) \bar{y} (λ) d λ}\} K_{m} \int_{Λ} L (λ) \bar{y} (λ) d λ .

The term in braces is a radiance-weighted integral of the beam attenuation coeﬃcient times the photopic luminosity function $\bar{y}$ . If we deﬁne the photopic beam attenuation coeﬃcient $c_{v}$ as

c_{v} (z, 𝜃, ϕ) \equiv \frac{K_{m} \int_{Λ} c (z, λ) L (z, 𝜃, ϕ, λ) \bar{y} (λ) d λ}{K_{m} \int_{Λ} L (λ) \bar{y} (λ) d λ},

(2)

then the $- c L$ term of the SRTE maintains the same form, $- c_{v} L_{v}$ , in the luminance transfer equation.

A similar treatment of the path radiance term of the SRTE leads to a deﬁnition for the photopic volume scattering function:

β_{v} (z, 𝜃^{'}, ϕ^{'} \to 𝜃, ϕ) \equiv \frac{K_{m} \int_{Λ} β (z, 𝜃^{'}, ϕ^{'} \to 𝜃, ϕ, λ) L (z, 𝜃^{'}, ϕ^{'}, λ) \bar{y} (λ) d λ}{K_{m} \int_{Λ} L (z, 𝜃^{'}, ϕ^{'}, λ) \bar{y} (λ) d λ} .

(3)

The source term in the SRTE leads to a photopic source term:

S_{v} (z, 𝜃, ϕ) \equiv K_{m} \int_{Λ} S (z, 𝜃, ϕ, λ) \bar{y} (λ) d λ .

Collecting the above results gives the desired luminance transfer equation:

\begin{align} cos 𝜃 \frac{d L_{v} (z, 𝜃, ϕ)}{d z} = & - c_{v} (z, 𝜃, ϕ) L_{v} (z, 𝜃, ϕ) \\ + & \int_{0}^{2 π} \int_{0}^{π} β_{v} (z; 𝜃^{'}, ϕ^{'} \to 𝜃, ϕ) L_{v} (z, 𝜃^{'}, ϕ^{'}) sin 𝜃^{'} d 𝜃^{'} d ϕ^{'} \\ + & S_{v} (z, 𝜃, ϕ) . & (4) \end{align}

This equation governs the propagation of broadband luminance as seen by the human eye in a one-dimensional absorbing and scattering medium. Equation (4) is the basis for the classical deﬁnition of contrast as used in visibility studies.

Dependence of $c_{v}$ on the Ambient Radiance

It is important to note that the photopic beam attenuation coeﬃcient as deﬁned in Eq. (2) depends on the ambient radiance distribution, hence on direction $(𝜃, ϕ)$ , even though the beam attenuation $c (z, λ)$ is an inherent optical property (IOP) that does not depend on the ambient radiance or direction. Moreover, $c_{v}$ meets the deﬁnition of an apparent optical property as deﬁned on the Apparent Optical Properties page: it depends on the IOPs of the medium (here the beam attenuation $c$ ) and on the ambient radiance distribution, and it is insensitive to external conditions (e.g., rescaling $L$ by a multiplicative factor does not change the value of $c_{v}$ ). The same holds true for the photopic volume scattering function deﬁned in Eq. (3) and for any other IOP. Thus, in going from a monochromatic radiative transfer equation to a luminance transfer equation, inherent optical properties become apparent optical properties. This is the penalty to be paid for going from an equation for monochromatic radiance as measured by instruments to an equation for luminance observed by a human eye.

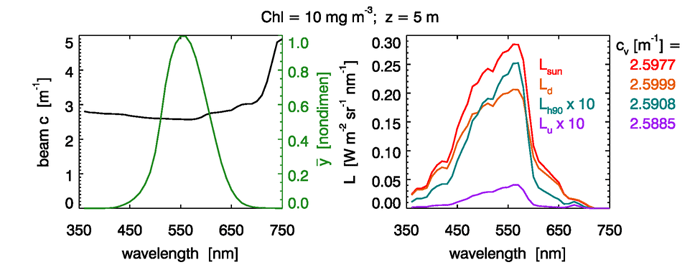
However, in practice, there seems to very little dependence of $c_{v}$ on the ambient radiance (as would be expected for a “good” AOP). The left panel of Fig. 1 shows the beam attenuation $c (λ)$ for a simulation of homogeneous Case 1 water with a chlorophyll concentration of $0.5 m g m^{- 3}$ (obtained using the new Case 1 IOP model in HydroLight). The Sun was at a zenith angle of $𝜃_{s u n} = 40 d e g$ in a clear sky, which gives a transmitted solar beam of about 29 deg in the water; that beam will lie in the HydroLight quad centered at $𝜃 = 30 d e g$ . The right panel of Fig. 1 shows the radiance at 10 meters depth looking in four directions: looking upward into the Sun’s transmitted beam, looking in the nadir and zenith directions, and looking horizontally at right angles to the solar plane.

The spectra in this ﬁgure were used to compute the photopic beam attenuation $c_{v}$ via Eq. (2). The values are all close to $0.31 m^{- 1}$ , which is close to the beam attenuation at the peak of the photopic luminosity function: $c (555 n m) = 0.313 m^{- 1}$ .

Figure 1: Left panel: Total (including water) beam attenuation

c (λ)

for a chlorophyll concentration of

0.5 m g m^{- 3}

(black curve), and the photopic luminosity function

ȳ

(green). Right panel: Radiances at a depth of 10 m for the Sun at a zenith angle of 40 deg in a clear sky.

L_{s u n}

(red curve) is looking upward into the Sun’s refracted beam.

L_{u}

(purple) is the upwelling (nadir-viewing) radiance;

L_{d}

(orange) is the downwelling (zenith-viewing) radiance; and

L_{h 90}

(green) is the horizontal radiance in the direction perpendicular (azimuthal angle of

ϕ = 90 d e g

) to the solar plane.

L_{u}

and

L_{h 90}

have been multiplied by 20 for better visibility in the plot. Numbers at the right show the photopic beam attenuation

c_{v}

for the four radiance spectra.

Figure 2 shows the corresponding results for a chlorophyll concentration of $10 m g m^{- 3}$ and a 5 m depth. Again, the four diﬀerent radiances give the same $c_{v}$ to within a fraction of a percent, and these $c_{v}$ values are within one percent of the beam attenuation value $c (555 n m) = 2.573 m^{- 1}$ .

Figure 2: Same as for Fig. 1, but for a chlorophyll concentration of

10 m g m^{- 3}

and a 5 m depth.

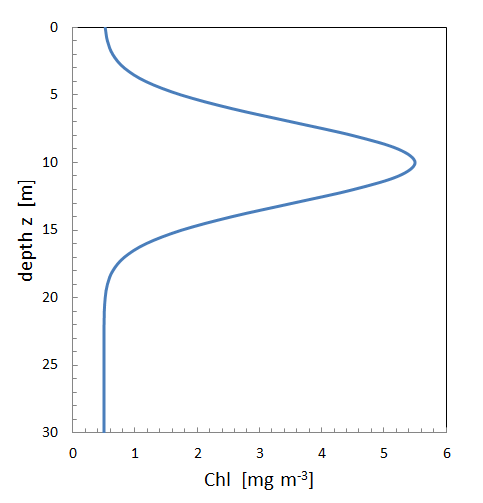
Figure 3 shows a chlorophyll proﬁle consisting of a background value of $0.5 m g m^{- 3}$ plus a Gaussian that gives a maximum value of $5.5 m g m^{- 3}$ at 10 m depth. For this proﬁle, an observer at 5 m depth looking upward would be looking into low-chlorophyll water, and looking downward would be looking into high-chlorophyll water. An observer at 10 m depth looking horizontally would be looking into high-chlorophyll water, but looking upward or downward would be looking into lower chlorophyll, clearer water. It might be supposed that the diﬀerent IOPs ( $c (z, λ)$ values in particular) would give radiances that might give signiﬁcantly diﬀerent $c_{v}$ values for the diﬀerent viewing directions at a given depth.

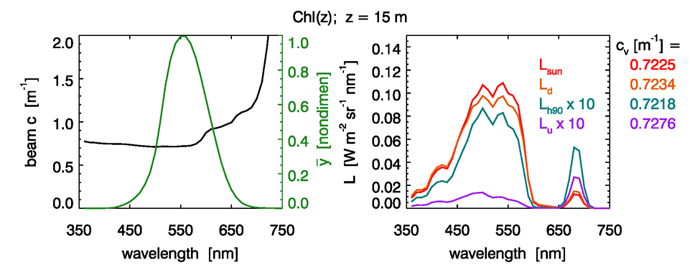
However, this is not the case. Figure 4 shows the radiances seen by an observer at 15 m depth. Again, the $c_{v}$ values diﬀer by only about one percent from the value of $c (15 m, 555 n m) = 0.719 m^{- 1}$ . The same holds true at other depths (not shown).

Figure 3: The chlorophyll proﬁle used in the simulations of Fig. 4.

Figure 4: Same as for Fig. 1, but for the depth-dependent chlorophyll proﬁle seen in Fig. 3 and a 15 m depth.

Exhaustive simulations have not been made, so it might be possible to create a contrived water body for which the photopic beam attenuation would be signiﬁcantly diﬀerent for diﬀerent viewing directions, and for which $c_{v} (z)$ would be signiﬁcantly diﬀerent from $c (z, 555 n m)$ . However, the above simulations indicate that in many situations of practical interest, there is little dependence of $c_{v}$ on viewing direction, and that $c_{v}$ is within a percent or so of the beam attenuation at the 555 nm wavelength of the maximum of the photopic luminosity function.

These simulations are consistent with the results of Zaneveld and Pegau (2003), who found that the beam attenuation coeﬃcient at 532 nm (excluding the water contribution) is a good proxy for the photopic beam attenuation.

See comments posted for this page and leave your own.

Comments for The Luminance Transfer Equation:

Loading Conversation

Contrast »

The Luminance Transfer Equation

The Luminance Transfer Equation

Dependence of cv on the Ambient Radiance

Comments for The Luminance Transfer Equation:

Dependence of $c_{v}$ on the Ambient Radiance